Hardy-Weinberg Problems

In class, 10/11/13, we learned the Hardy- Weinberg formula, which determines the frequency of alleles(genes) in a population. Hardy and Weinberg argued that if five conditions are met, the population's allele and genotype frequencies will remain constant from generation to generation. The five conditions are:

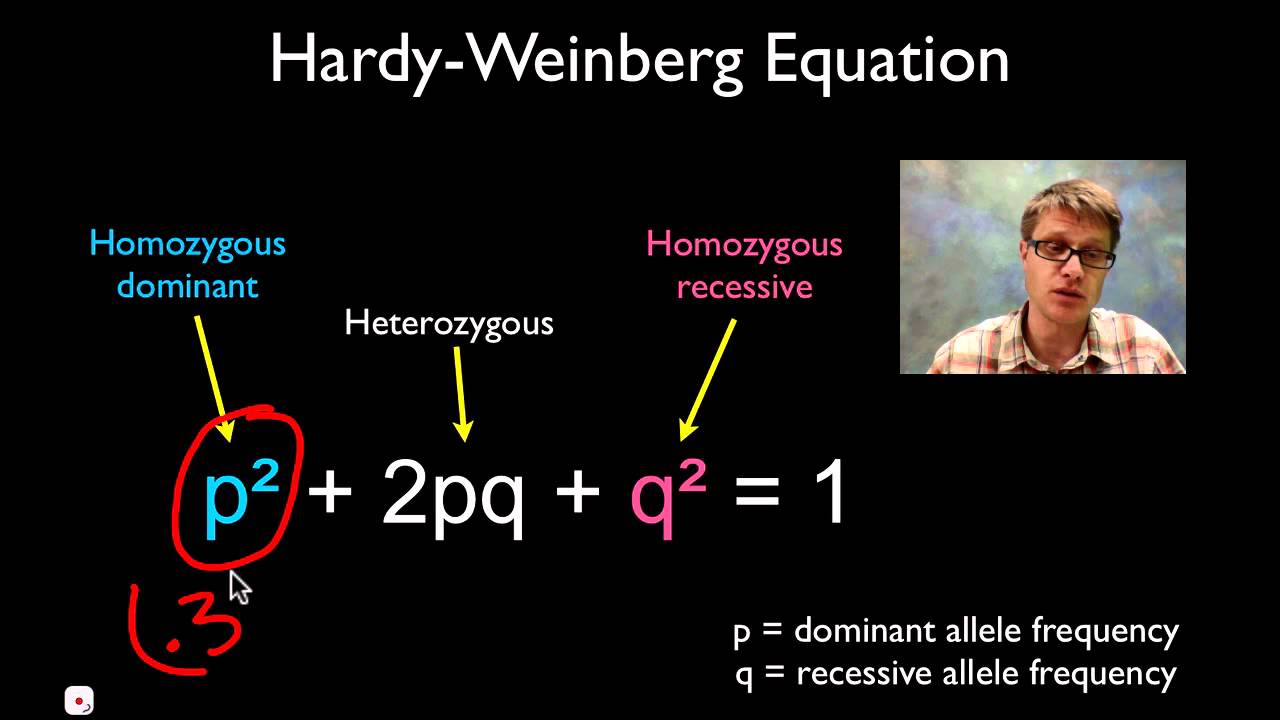
The Hardy-Weinberg formula is:



Here is a Hardy- Weinberg Problem:

 In certain African countries 4% of the newborn babies have sickle-cell anemia, which is a recessive trait. Out of a random population 1,000 newborn babies, how many would you expect of the three possible genotypes?

The first thing you look for is a q(recessive allele frequency) or q^2 (homozygous recessive). The q^2 in this problem is 4% of newborn babies. In order to find q you mush square root the 4%. So the value of q is 0.2. To find p you can just subtract 1-0.2, since you know that p+q=1. p=0.8, and q=0.2.

The problem also asks for the three possible genotypes (q^2, p^2, and 2pq). In order to find these values, you must multiply  your values for q^2, p^2 and 2pq by the total population, 1000.

p=0.8

q=0.2

2pq=0.32

population=1000



Now we use these values to find the number of individuals in each genotype for this population

q^2*population=number of individuals with recessive trait(sickle-cell anemia)

(0.04)^2*1000=40 individuals

p^2*population=number of individuals with the dominant trait

(0.8)^2*1000=640 individuals

2pq*population= number of individuals with the heterozygous trait

(0.32)*1000=320 individuals